Recently I watched a lecture by Elizabeth Spiegel online where she talked about the importance of blunder-checking, especially for scholastic players. It’s not a new idea. Before you make your move, she said, write it down, and then look back at the board and ask, “Can my opponent take this piece?” After you’re sure that she cannot take the piece you’re planning to move, then you can go ahead and play the move, and put a check mark by it. The check mark indicates that you’ve performed the blunder check.
“This technique will not work without the check mark,” she stressed. Otherwise the student will forget to blunder-check, or she will say that she did when she really didn’t. But the act of having to make the check mark, and thinking about what it means, will force her out of whatever thought pattern she was in before.
I’m mentioning this in my blog, firstly because some of my readers might want to try it. Secondly, I wonder whether there is a possible extension of this technique (perhaps suitable for more advanced players). It’s called the brilliancy check.
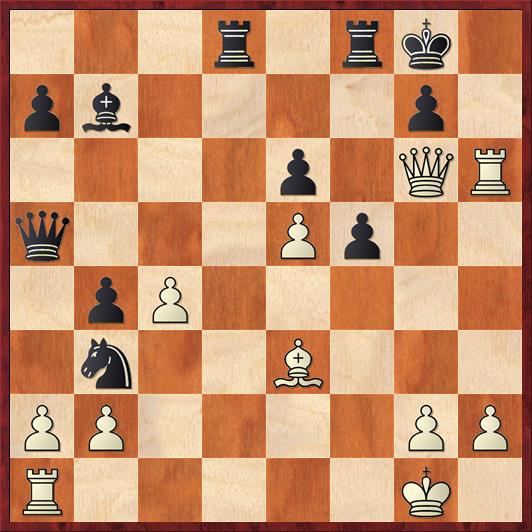
FEN: 3r1rk1/pb4p1/4p1QR/q3Pp2/1pP5/1n2B3/PP4PP/R5K1 w – – 0 28
Here is a prototypical example, from a game I played against the computer (Shredder engine, set at a strength of 2288). When I got to this position I thought that I was just going to play 28. Qh7+ Kf7 29. Qg6+ Kg8 and repeat the position. But then a funny thing happened. I accidentally noticed that the machine was evaluating this position at +17.30 pawns for me. (Usually I try my best to avoid looking at the machine’s evaluation, because I think it’s a form of cheating.)
If the machine says it’s +17.30, that means it’s a dead certainty that White has some tactical shot. And sure enough, it didn’t take me long to spot it: 28. Qh7+ Kf7 29. Rf6+! This forces the king away from the g-pawn, and he is left with no shelter. After 29. … Ke8 30. Qxg7! (much better than 30. Qg6+) White threatens mate on f8. Black is forced to play 30. … Rxf6 31. ef. Now mate is threatened on e7, and there is no real way to stop it. (The move 31. … Rd7 allows the pretty 32. Qg8 mate.) So Black resigned.
But what if I hadn’t seen the evaluation, which was the equivalent of the computer telling me, “White to play and win”? How could I have slowed myself down and forced myself to look for something better than a draw by repetition?
Okay, first, let me say that if it were a tournament game and I had plenty of time on my clock, I think I would have eventually hit upon the idea of 30. Rf6+. Probably. Nine times out of ten. But weird things do happen sometimes, and I’m sure that I have missed some equally simple wins in my life.
In order to spot something like this, I would like to propose a new technique called “brilliancy checking.” You decide on what you’re going to play, write it down (if you like that technique), and then you ask,
“If someone told me that this position was White to play and win, would I still play this move?”
Most of the time, your conclusion will be that the person who said that was nuts. But now and then, maybe one game in ten or one game in fifty, you might find that you have a winning move.
Of course, this is only a proposal. I haven’t tried it yet in an actual game. I just played the above game against the computer today. But if any of my readers want to try it, please tell me whether “brilliancy checking” works. And if so, send me an example!
P.S. There’s another psychologically interesting thing about this example — the way that the moves 28. Qh7+ Kf7 trade a vertical pin on the g-pawn for a horizontal pin. Somehow the horizontal pin was harder to spot, and this explains why I didn’t originally see the idea of 29. Rf6+. Perhaps subconsciously, when I moved my queen to h7, I thought, “I am releasing the pin on the g-pawn,” and then after 29. … Kf7 it didn’t occur to me that the pin was restored.
It’s always tricky to spot and explain faulty thought patterns. When you bring them out into the light they always turn to dust and blow away, because they are so obviously wrong. But that makes it even more important to try to understand the irrational belief or faulty thought pattern that caused you to make your mistake.

