One of the first things that kids love to do, after they learn the rules of chess, is to tamper with the rules. I’m not sure why. For example, they say, “Let’s make every piece a queen!” Sounds like fun in principle, but what happens in practice is that every move is a capture and there is essentially no strategy involved. It’s actually quite hard to improve on the rules of chess. The rules we have do a very nice job of balancing artistry and competitiveness.
However, one undeniable inequity in chess is the fact that White wins more often than Black. Tournament directors, for example, have to go to great lengths to avoid giving players too many Blacks, and they can’t always succeed.
This week I was interested to discover a proposal to remedy this imbalance, which was devised not by a kid at chess club but by two game theorists, Steven Brams of New York University and Mehmet Ismail of King’s College London. The idea of what they call Fairer Chess is very simple: White starts with one move as usual. Then Black plays two consecutive moves. Then White plays two consecutive moves. Then the players play normally for the rest of the game. You can read more about Brams and Ismail’s work here.
The two researchers have done this sort of thing before. In an earlier paper called Making the Rules of Sports Fairer, they argue that penalty-kick shootouts in soccer are biased in favor of the first team. On the other hand, in tennis tiebreakers, one player serves once, then the second player serves twice and the two players continue to serve twice in a row. Brams and Ismail show that this rule does not give either player an advantage.
Of course, if we implemented this rule in chess we would do violence to the game, because moving twice in a row fundamentally changes the strategy of the game (as I’ll discuss below). The really innovative thing about Brams and Ismail’s paper is that they claim that just one round of double-moves of Black and one for White is sufficient to even out the odds. Ismail says that a 47-ply analysis by Stockfish gave an evaluation of 0.0 for this game.
Is it true that this one tiny alteration would make chess fairer? I have to admit, I was skeptical. But one nice thing about their proposal is that it should be possible to refute or prove this claim by direct analysis. When I did this, I discovered something quite interesting — an unintended consequence of the new rule that, in my opinion, makes Fairer Chess aesthetically less satisfying, even if it is fairer.
As an e4 player, I naturally started by asking, “How does Black answer 1. e4?” But then I realized that an even more critical question is: “How does Black answer 1. d4?”
As we know, the chess world falls almost entirely into two camps: the Classical camp that plays 1. … d5, and the Indian camp that plays 1. … Nf6, leading usually to the King’s Indian (2. … g6) or the Nimzo-Indian (2. … e6).
What I realized is that both of these Indian move orders are bad in Fairer Chess. If Black tries for a King’s Indian setup, with 1. d4 (Nf6, g6), then White responds with the kamikaze bishop maneuver 2. (Bh6, Bxf8). Black is deprived of the castling privilege and also his kingside is notably weakened by the exchange of bishops. This cannot be good for Black.
Likewise the Nimzo-Indian setup works out badly. After 1. d4 (Nf6, e6), White can play another kamikaze attack, 2. (e4, e5). After 2. … Nd5 Black is not in a Nimzo-Indian but a markedly inferior variation of Alekhine’s Defense, where White can either play 3. Nf3 without fear of … Bg4 or he can play 3. c4 without fear of an eventual … Bf5.
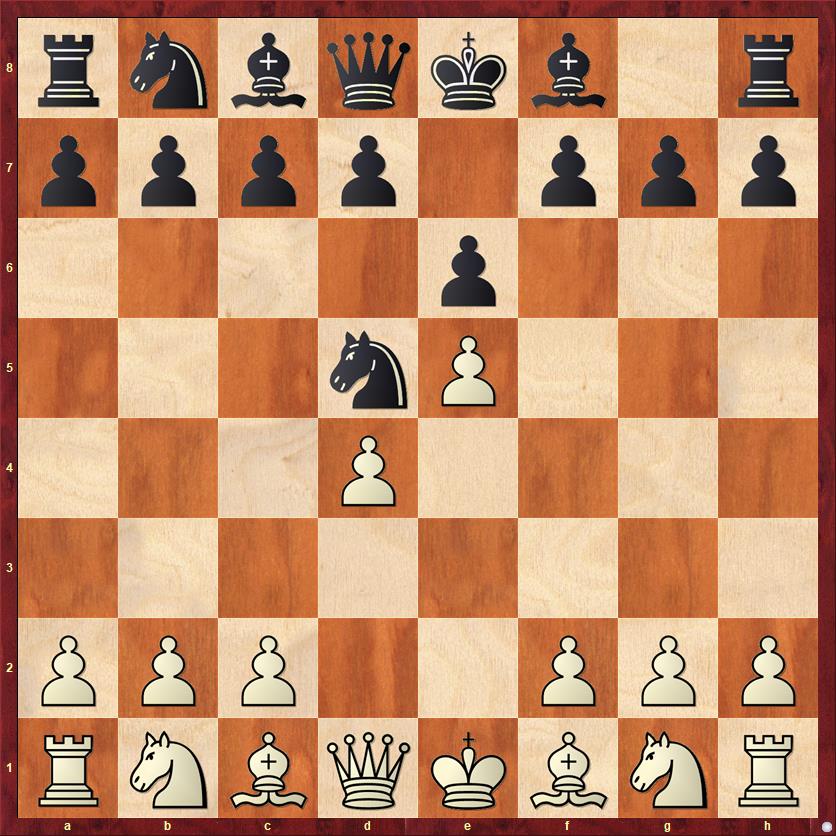
rnbqkb1r/pppp1ppp/4p3/3nP3/3P4/8/PPP2PPP/RNBQKBNR w KQkq – 0 4
If Black really wants to try for an Indian setup, I think he has to play 1. d4 (Nf6, d6). But then White does not have to go into a King’s Indian; he can go into a Pirc instead with 2. (e4, Nc3). Although I could be wrong, I don’t think that the Pirc commands quite the respect among chess players that the King’s Indian does, and there are a lot of King’s Indian players who would not be completely happy at being forced to play the Pirc.
What about our Classical players? For aficionados of the Queen’s Gambit Declined, the news is even worse: After 1. d4 (d5, e6), White can win Black’s queen with the kamikaze moves 2. (Bg5, Bxd8). Horrors!
In fact, the only Classical setup that seems completely sound to me in Fairer Chess is the Slav-style 1. d4 (d5, c6). Now White can choose between 2. (e4, xxx) going into a Caro-Kann or 2. (c4, xxx) going into a Slav.
A final possibility for Black is to anticipate White’s kamikaze attacks by playing a kamikaze of his own on the first move. There are two possibilities. Extremely bad is 1. d4 (e5, exd), when White again wins the queen with (Bg5, Bxd8). But the other possibility, 1. d4 (c5, cxd) is probably Black’s best response to 1. d4. Once again White’s main choices seem to be going into an Exchange Slav with 2. (c3, cxd) or an Exchange or Panov Caro-Kann with 2. (e3, exd). There is also a possibility of going into a Sicilian with 2. (e4, Nf3), which could lead to some interesting Smith-Morra gambit play because Black can play 2. … e5. White can’t take because of 3. Nxe5?? Qa5+ and has to continue in gambit style with 3. c3 or 3. Bc4.
So, to sum up our investigation:
- Fairer Chess turns opening theory back nearly 100 years by making the most popular Indian variations (King’s Indian and Nimzo-Indian) clearly weaker than before (assuming you agree that the Pirc is not quite as strong as the King’s Indian).
- Fairer Chess also kills the Queen’s Gambit Declined.
- For Black, the only good thing about Fairer Chess is that people who like the Slav and the Caro-Kann can get the type of position they like. In fact, they can direct play into variations of these openings where White exchanges on d5.
Do these changes make chess fairer? In fact, they do, by a tiny amount. According to ChessBase, in master games White scores about 55 percent. But in the Exchange Slav, the Exchange Caro-Kann or the Panov Caro-Kann White scores only 52 or 53 percent. Likewise, in the Smith-Morra-like 1. d4 (c5, cxd) 2. (e4, Nf3) White scores about 53 percent, but with a small sample size.
But this statement comes with a big asterisk. The cost of making chess fairer in this way is that we cripple the Indian defenses. More generally, I think the researchers fell into a fallacy that many chess players do, as well. When you see that the computer evaluates a position at 0.0, you think, “Oh, it’s even.” But it makes a big difference how broad the spectrum of choices is. If your opponent has to find one precise sequence of moves to equalize, and otherwise he’s lost, I would argue that the position is not really equal. In fact, AlphaZero (Google’s chess program) is able to detect this difference, because it evaluates positions based on the probability of winning rather than by an abstract calculation involving tenths or hundredths of a pawn.
We have a similar phenomenon here. Black’s range of options has decreased considerably, so that if he wants to benefit fully from Fairer Chess’s fairness, he has to study the Slav Defense and the Caro-Kann Defense. I think this is kind of sad. One of the beauties of normal chess is that there is such a huge variety of openings and defenses, and hardly any of them have ever been refuted! This makes it possible to choose a defense based on your personal taste. In Fairer Chess, I fear, that is not the case.
Is there any way to rescue Fairer Chess? One idea that occurred to me would be another rule: when you play two moves in a row, they have to be with different pieces. That prevents the “kamikaze variations,” especially the ones with (Bg5, Bxd8) and (Bh6, Bxf8) that penalize Black so severely for a move that shouldn’t really be a mistake. However, this anti-kamikaze rule might also weaken the equalizing effect of the double-moves. Perhaps, to get the full effect, you would have to keep the alternation going a little bit longer, say for another pair of double moves.
Finally (and I hope I’m not being too picky) is this problem really a problem? As a spectator, watching games at the GM level, I like the fact that White has a slight advantage. It means that a championship match is not uniformly in favor of the stronger player. The weaker player will also have a chance to win now and then, and the stronger player will have to show his skill at defending as well as attacking. And at the amateur level. the disparities in playing strength are almost always greater than the advantage of playing White.
What do you all think? I’ve been in touch with Ismail and Brams, and I’m sure that they would be interested in feedback from real chess players.
