For the Aptos Library Chess Club this week I wanted to teach a lesson based on one of Sam Shankland’s games from the recently concluded U.S. Championship. I think that the kids should know who the best players are … especially when one of them came from our area and not too long ago was just like them.
First question: Which game? I had watched his games against Lenderman and Liang live and didn’t think there were any great teachable moments in those games. Then I looked at his win against Robson, and it also had no spectacular turning points; Robson just lost a pawn and got ground down.
Then I played over the game Izoria-Shankland and I knew I had a lesson. Here is how it ended.
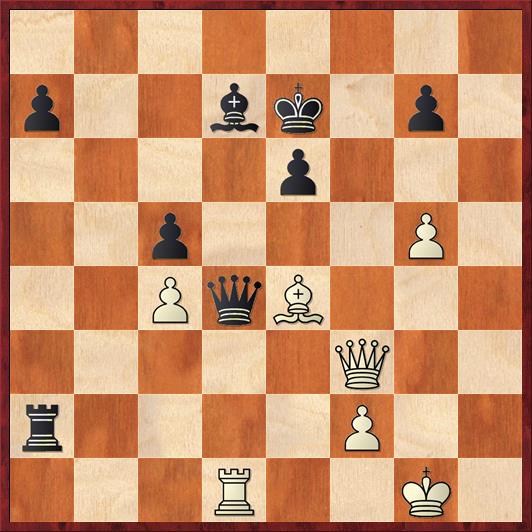
Position after 1. Rd1. Black to move.
FEN: 8/p2bk1p1/4p3/2p3P1/2PqB3/5Q2/r4P2/3R2K1 b – – 0 1
Here Shankland plays a move that is good but not that hard to spot. It’s the same move I would have played.
1. … Ra1!
There are some good lessons here about not being seduced by the chance to win material. Black could grab a pawn with 1. … Qxc4, but he loses the game after the tricky 2. Qf4! The threat here is to play 3. Rxd7+! followed by 4. Bc6+ with a discovered attack on Black’s queen. Also, White threatens to penetrate Black’s position in a damaging way with either 3. Qd6+ or 3. Qc7. Black cannot defend all of these threats, and in fact the computer shows White with a 1.5-pawn advantage.
But even without working out all these tactics, the move 1. … Qxc4 doesn’t “feel right.” Black is already a pawn up, and the biggest concern in this position is that his king is caught between the crossfire of White’s rook on the d-file and queen on the f-file. In such a position it cannot be right to turn the initiative over to the opponent. Instead, 1. … Ra1! keeps the initiative in Black’s hands — the threat is to win the rook on d1. It defends a threat with a threat. And also, even a rook trade (1. … Ra1 2. Rxa1 Qxa1+) is fine for Black because we’re already a pawn up, and this endgame should be winning (although it may take a while). It’s especially important to realize that, after a rook trade, there is no longer any possibility of a White win, as there would have been after 1. … Qxc4. We are “playing for two results” rather than three.
This is an interesting case where understanding the strategy saves you from having to navigate through tricky tactics.
White, naturally, does not want to trade rooks, so he plays
2. Bb1 …
and now Sam comes up with a really nice winning move.
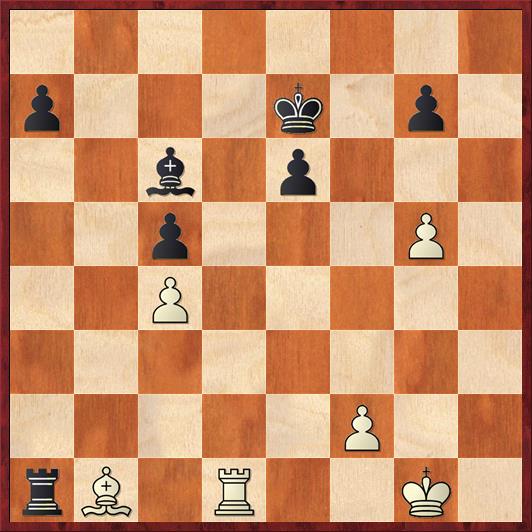
Position after 2. Bb1. Black to move.
FEN: 8/p2bk1p1/4p3/2p3P1/2Pq4/5Q2/5P2/rB1R2K1 b – – 0 2
2. … Bc6!
There are so many points to this move that it’s hard even to list them all, but Black wins either a pawn or the exchange in all variations, leaving White completely busted. For example:
(a) 3. Qxc6 Qxd1+ is the most obvious point. A deflection.
(b) 3. Rxd5 Bxf3 is really cute — at first sight it’s just a queen trade, but then you see that White has two pieces en prise and he can’t defend them both.
(c) Otherwise, White must move his queen to a square that guards the rook. 3. Qg4? Qxg4+ is an obvious loser.
(d) A better try is 3. Qh5, but it has a neat refutation: 3. … Rxb1! 4. Rxb1 Qe4, threatening both mate on g2 and the rook on b1. Also we must pause to admire how the bishop prevents any zwischenzug checks.
(e) Probably White’s best move is 3. Qe2, but now Black has 3. … Qh4! which threatens mate on h1. The only way out is for White to block the diagonal with 4. f3. Interestingly, the kids pointed this out and I had not thought about what Black’s best response is! In chess club I said 4. … Qxg5+, which is certainly good, but I think that 4. … Qg3+ is probably even better, because it both wins the f3 pawn and trades queens, leading to an easy endgame win.
(f) Finally there is 3. Qb3, which we somehow missed in chess club, but Black has a really cool response here, too: 3. … Ra3! The point now is to mate on h1 with the rook instead of the queen. So, for example, 4. Rxd4 Rxb3 5. Rd1 Rh3! forces White to give up a pawn or an exchange (e.g., 6. Kf1 Rh1+ 7. Ke2 Bf3+). And 4. Qc2 allows Black to offer a queen sacrifice with 4. … Rh3! Of course White can’t take it, and this time he can’t even play f3 to block the diagonal, so 5. Kf1 is forced and then 5. … Bg2+! is the coup de grace. White is mated after 6. Kxg2 Qg4+ and he loses a bunch of material if he plays 6. Ke2 or 6. Ke1.
Awesome stuff! I just love all the different directions Black’s pieces go in, and the sneaky way in which the more “harmless”-looking variations turn out deadly for White. Izoria chose none of the above variations: he simply resigned.
Now, let’s think: How do we turn this into a lesson? First, you have to realize who your audience is. I would have loved to talk about the position on move 1, but my audience of kids and beginners is not going to understand the subtle points of strategy versus tactics. Not only that, if we begin on move 1 we will have to spend ten minutes talking about that position, which leaves at best five or ten minutes for talking about the position on move 2. That’s just not enough because of all the non-obvious moves that the kids have to find.
So the first thing I decided was to start with move two. But even this is not easy because of the multiple variations. How do we make it easier for the kids? That’s when I had my best idea. Let’s not start with this position. Instead, let’s take the queens off the board and move Black’s bishop to c6:
Black to play and mate in zero.
FEN: 8/p3k1p1/2b1p3/2p3P1/2P5/8/5P2/rB1R2K1 b – – 0 0
Here, instead of asking the kids, “What should Black’s move be?” I asked them, “Suppose Black had a queen and could put it anywhere on the board. Where would you place it to create a checkmate?” We can call this a “Black to play and mate in zero” problem.
The great thing about starting this way is that, first of all, we work on “in your face checkmates” all the time. So the kids had no trouble spotting 0. … Qh1 mate and 0. … Qg2 mate. (I was surprised, though, that they saw the one on h1 first.) It’s important to start the lesson with an easy question that they can answer. But also, I think it’s important because I strongly suspect that the checkmates are what Sam saw first in the position. They are the nuclear energy that powers the rest of the combination. The way you find the moves 3. … Qh4 (in variation e) and 3. … Rxb1 followed by 4. … Qe4 (in variation d) is by looking for the checkmates.
I regret only that I did not also ask them, “If you could move Black’s rook to any other square on the board, where would you put it to get a checkmate?” Then they would have found 0. … Rh1 mate, and we would have been ready to find the solution to variation f as well.
After that we went back to the position on move 2, and then the difficult-to-find move 2. … Bc6 became blindingly obvious. One kid did want to play 2. … Qxc4, which may actually be okay here because White no longer has his tactical trick with Qf4. But once you’ve seen 2. … Bc6 it’s clearly a better way to go. All in all, I was really satisfied with the way that the “mate in zero” problem prepared them to navigate the complexities of variations a through e. I’m only kicking myself for missing variation f.
Finally, one interesting thing about working with kids is the questions you weren’t expecting. I had put up cards next to the board with the names of the players. One girl raised her hand, when we were about ten minutes into the lesson, and asked, “Could you pronounce the White player’s name?”
Everybody laughed. Her question made me uncomfortable, because I felt there was an implication that a name like Zviad Izoria is weird. We have a kid in the chess club whose first name is Reza. Is that weird, too? In Donald Trump’s America, are people with funny names not welcome?
I just decided to let them have their laugh and move on. But maybe I blew it. Maybe I should have told them, Zviad Izoria came to this country from Georgia (can you find Georgia on a map?) because he wanted to have greater opportunities. I actually played in his first American tournament, the HB Global Challenge in 2005, where he won $50,000, which would have been several years of income for a chess player in Georgia. This year he played in the U.S. championship for the first time, and he had a great tournament, with wins over Fabiano Caruana and Hikaru Nakamura. His win over Caruana was arguably the most important game of the tournament: Caruana won six games but lost one, while Shankland won six games without losing any. That was the margin of difference between them.
Perhaps I should make up for this by showing them the game Caruana-Izoria next week. Or do you think I’m making a big deal out of nothing, and should just let it slide?



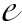
{ 5 comments… read them below or add one }
I wish you would’ve refrained from a political reference in this article.
The tag “Donald Trump” does not belong to it, remember the kids come from the Santa Cruz county, and the last person they get influenced by there is Donald Trump.
A propos, your name is funny to every Hebrew ear, in Israel Dana is a girl’s name.
Exactly the point. Everybody’s name sounds funny to someone somewhere. I may be reading too much into the girl’s question, because maybe she really just did want to know how to pronounce it. But the way the other kids reacted definitely made me think that they were laughing at Zviad Izoria because his name sounded funny.
Well, names are fun. They`re supposed to be! Donald Trump, too.
But if this “In Donald Trump’s America, are people with funny names not welcome?” is the actual criticism about the state of your country and/or the leadership of it, you are doing really well. As you seem to be (compared to Europe..) Congrats.
Nice article and a great illustration of how important tactics are in all phases of the game. Sometimes players think that tactics are important and pretty only when there are tons of pieces left. But with fewer pieces (as here), the extraneous complexity is stripped away and the pure geometry of these line-moving pieces gets emphasized.
Another good recent example is from Bluebaum-Vitiugov in the first round of the recent Grenke tournament. There, just as here, it’s not just the initial moves that are instructional, but the side variations and vain attempts to defend. Of course, one doesn’t know these defensive measures are in vain until an exhaustive analysis is completed, which is the ultimate point of analysis.
Re: name question. I would like to think it was an innocent/good faith question, and that the laughter was due to its apparent irrelevance to the lesson. I wasn’t there, of course, and so can’t begin to say.
But, as you suggest, maybe it was indeed a “teachable moment” (not my favorite phrase, but apt). It would have been cool had you known that “Zviad” means “proud, arrogant” in Georgian and been able to point that out (maybe leaving out “arrogant”!), along with your personal experience and knowledge of him.
Along those lines, the name of your student “Reza” is commonly pronounced with either a short or a long e sound. Also, it’s quite fair to wonder how the US Women’s Champion Paikidze pronounces her first name. Chess has such a rich history when it comes to the names of its players, openings, and tournaments that these names deserve to be reveled in.
Paikidze pronounces her name as “Nuh-Zee”. That “controversy” was mentioned in one of the recent (2016) Chess Life issues.